Formula For Finding Volume Chemistry

Ideal gas law or perfect gas law represents the mixed relationship between pressure, volume, the temperature of gases for learning the physical properties of the gas molecule in physics or chemistry. The ideal gas equation balancing these state variables in terms of universal gas constant . The ideal or perfect gas law formula can use for calculating the value of pressure, volume, temperature, diffusion or effusion, concentration, and the number of gas molecules per unit volume or density.

Boyle's in 1662, Charles's in 1787, and Avogadro law give the general derivation formula of the ideal or perfect gas equation, and the kinetic theory of gas provides the properties of ideal gases. Eventually, these individual laws were combined into a single equation—the ideal gas law—that relates gas quantities for gases and is quite accurate for low pressures and moderate temperatures. We will consider the key developments in individual relationships , then put them together in the ideal gas law. The behavior of gases can be described by several laws based on experimental observations of their properties.

The pressure of a given amount of gas is directly proportional to its absolute temperature, provided that the volume does not change (Amontons's law). The volume of a given gas sample is directly proportional to its absolute temperature at constant pressure (Charles's law). The volume of a given amount of gas is inversely proportional to its pressure when temperature is held constant (Boyle's law). Under the same conditions of temperature and pressure, equal volumes of all gases contain the same number of molecules (Avogadro's law). And is a proportionality constant that relates the values of pressure, volume, amount, and temperature of a gas sample.

The variables in this equation do not have the subscripts i and f to indicate an initial condition and a final condition. The ideal gas law relates the four independent properties of a gas under any conditions. Therefore, the unit that results from the division of the indicated quantities is "g/mL," which is a unit that is typically utilized to report thedensityof a substance.

However, as stated previously, the quantity of solute that is present in a given solution can be expressed using three unique percent-based concentrations. Gases whose properties of P, V, and T are accurately described by the ideal gas law are said to exhibit ideal behavior or to approximate the traits of an ideal gas. An ideal gas is a hypothetical construct that may be used along with kinetic molecular theory to effectively explain the gas laws as will be described in a later module of this chapter.

Although all the calculations presented in this module assume ideal behavior, this assumption is only reasonable for gases under conditions of relatively low pressure and high temperature. In the final module of this chapter, a modified gas law will be introduced that accounts for the non-ideal behavior observed for many gases at relatively high pressures and low temperatures. The volume and temperature are linearly related for 1 mole of methane gas at a constant pressure of 1 atm.

If the temperature is in kelvin, volume and temperature are directly proportional. Charles's law states that the volume of a given amount of gas is directly proportional to its temperature on the kelvin scale when the pressure is held constant. Therefore, only the equation that is shown above can be applied to reliably determine the mass/volume percent of a solution. This relationship between temperature and pressure is observed for any sample of gas confined to a constant volume. An example of experimental pressure-temperature data is shown for a sample of air under these conditions in .

Volume is the quantification of the three-dimensional space a substance occupies. By convention, the volume of a container is typically its capacity, and how much fluid it is able to hold, rather than the amount of space that the actual container displaces. Volumes of many shapes can be calculated by using well-defined formulas. In some cases, more complicated shapes can be broken down into simpler aggregate shapes, and the sum of their volumes is used to determine total volume. The volumes of other even more complicated shapes can be calculated using integral calculus if a formula exists for the shape's boundary. Beyond this, shapes that cannot be described by known equations can be estimated using mathematical methods, such as the finite element method.

Alternatively, if the density of a substance is known, and is uniform, the volume can be calculated using its weight. This calculator computes volumes for some of the most common simple shapes. An example of experimental pressure-temperature data is shown for a sample of air under these conditions in Figure 9.11. Answer 1) The answer to this question depends on how we define concentration.

If we take concentration by mass into consideration, it will still change, unless the substance is with an undefined density. That's because the mass of a substance will change with its volume, and so the concentration changes. But, if both the solute and solvent are either increasing or decreasing in volume/mass/moles in an equal ratio, the concentration and molarity will remain the same. To apply this gas law, the amount of gas should remain constant. As with the other gas laws, the temperature must be expressed in kelvins, and the units on the similar quantities should be the same.

Because of the dependence on three quantities at the same time, it is difficult to tell in advance what will happen to one property of a gas sample as two other properties change. If a sample of gas has an initial pressure of 1.56 atm and an initial volume of 7.02 L, what is the final volume if the pressure is changed to 1,775 torr? Assume that the amount and the temperature of the gas remain constant. For example, the space that a substance or 3D shape occupies or contains.

Volume is often quantified numerically using the SI derived unit, the cubic metre. Volumes of some simple shapes, such as regular, straight-edged, and circular shapes can be easily calculated using arithmetic formulas. Volumes of complicated shapes can be calculated with integral calculus if a formula exists for the shape's boundary. One-dimensional figures and two-dimensional shapes are assigned zero volume in the three-dimensional space. Is the volume occupied by one mole of a chemical element or a chemical compound. It can be calculated by dividing the molar mass by mass density (ρ).

Molar gas volume is one mole of any gas at a specific temperature and pressure has a fixed volume. Ideal gas law defines the relation between pressure, volume, temperature, and composition of gases. But the equation is found to hold most satisfaction when pressure is low or tense to zero. At ordinary temperature and pressure, the equation is found to deviated about 5%. Therefore, the real or Van der Waals gas obeys ideal gas law only at low pressures and very high temperatures. These calculations are especially useful when working with compounds that do not have well-defined molecular weights .

This calculator finishes the topic started in Convert moles to liters and liters to moles calculator. Because the molar volume is the same for all ideal gases and is known, we can convert from grams to liters and vice versa if we know the gas formula. Avogadro was an Italian Physicist who first described the Avogadro constant as a hypothesis in 1811. He was trying to understand why in chemical reactions involving gases the observation that equal volumes of different gases had the same number of moles.

This was found true even when the masses were very different. The idea that a mole of any substance has exactly the same number of atoms no matter what the substance is made of was explained by Avogadro and his name has stuck to his number ever since. If a sample of gas has an initial pressure of 375 torr and an initial volume of 7.02 L, what is the final pressure if the volume is changed to 4,577 mL? Assume that amount and the temperature of the gas remain constant. In thermodynamics, the volume of a system is an important extensive parameter for describing its thermodynamic state.

The specific volume, an intensive property, is the system's volume per unit of mass. Volume is a function of state and is interdependent with other thermodynamic properties such as pressure and temperature. For example, volume is related to the pressure and temperature of an ideal gas by the ideal gas law. Experience has shown that several properties of a gas can be related to each other under certain conditions. The properties are pressure , volume , temperature , and amount of material expressed in moles .

What we find is that a sample of gas cannot have any random values for these properties. Instead, only certain values, dictated by some simple mathematical relationships, will occur. It is important to note that the molarity is defined as moles of solute per liter of solution, not moles of solute per liter of solvent. This is because when you add a substance, perhaps a salt, to some volume of water, the volume of the resulting solution will be different than the original volume in some unpredictable way. To get around this problem chemists commonly make up their solutions in volumetric flasks.

These are flasks that have a long neck with an etched line indicating the volume. The solute is added to the flask first and then water is added until the solution reaches the mark. The flasks have very good calibration so volumes are commonly known to at least four significant figures.

An aqueous solution consists of at least two components, the solvent and the solute . Usually one wants to keep track of the amount of the solute dissolved in the solution. One could do by keeping track of the concentration by determining the mass of each component, but it is usually easier to measure liquids by volume instead of mass. Molarity is defined as the number of moles of solute divided by the volume of the solution in liters. This means equal amounts of moles of gases occupy the same volume under the same conditions of temperature and pressure.

The ideal gas equation contains five terms, the gas constant R and the variable properties P, V, n, and T. Specifying any four of these terms will permit use of the ideal gas law to calculate the fifth term as demonstrated in the following example exercises. Temperature is sometimes measured with a gas thermometer by observing the change in the volume of the gas as the temperature changes at constant pressure. The hydrogen in a particular hydrogen gas thermometer has a volume of 150.0 cm3 when immersed in a mixture of ice and water (0.00 °C). When immersed in boiling liquid ammonia, the volume of the hydrogen, at the same pressure, is 131.7 cm3.

Find the temperature of boiling ammonia on the kelvin and Celsius scales. Answer 2) First step is to multiply the molarity by the molar mass of the solute to get grams of solute per litre. The second step is to divide the concentration expressed as grams of solute per litre by the density of the solution in grams per litre. Finally, multiply it by 100% to convert it to percentage.

Imagine filling a rigid container attached to a pressure gauge with gas and then sealing the container so that no gas may escape. If the container is cooled, the gas inside likewise gets colder and its pressure is observed to decrease. Since the container is rigid and tightly sealed, both the volume and number of moles of gas remain constant.

If we heat the sphere, the gas inside gets hotter () and the pressure increases. What is its volume if the temperature is changed to −35°C? Assume that the pressure and the amount of the gas remain constant. What is its volume if the temperature is changed to 60°C?

If a sample of gas has an initial pressure of 3.66 atm and an initial volume of 11.8 L, what is the final pressure if the volume is reduced to 5.09 L? If a sample of gas has an initial pressure of 1.56 atm and an initial volume of 7.02 L, what is the final volume if the pressure is reduced to 0.987 atm? Examples and practice problems of solving equation stoichiometry questions with gases. We calculate moles with 22.4 L at STP, and use molar mass and mole ratios to figure out how many products or reactants we have. The most common molar volume is the molar volume of an ideal gas at standard temperature and pressure (273 K and 1.00 atm).

The line stops at 111 K because methane liquefies at this temperature; when extrapolated, it intersects the graph's origin, representing a temperature of absolute zero. If we heat the sphere, the gas inside gets hotter (Figure 9.10) and the pressure increases. If we heat the sphere, the gas inside gets hotter and the pressure increases.

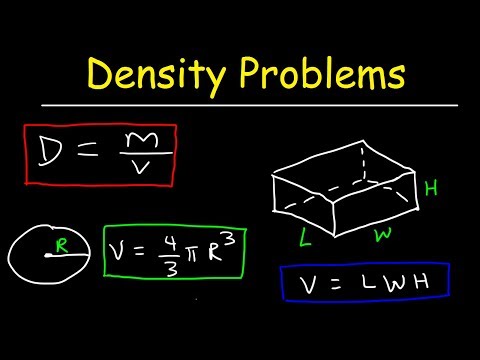
Of a substance is the ratio of the mass of a sample of the substance to its volume. The SI unit for density is the kilogram per cubic meter (kg/m3). For many situations, however, this as an inconvenient unit, and we often use grams per cubic centimeter (g/cm3) for the densities of solids and liquids, and grams per liter (g/L) for gases. Although there are exceptions, most liquids and solids have densities that range from about 0.7 g/cm3 to 19 g/cm3 .

Table \(\PageIndex\) shows the densities of some common substances. In ideal gas law, the volume is an intensive property but temperature, pressure are extensive properties in thermodynamics derivation. The four thermodynamics variables in the gas laws for the ideal or perfect gases are pressure, volume, temperature, and mole number.

Some of these depend on the mass of the system while others are independent of the mass. If we partially fill an airtight syringe with air, the syringe contains a specific amount of air at constant temperature, say 25 °C. This example of the effect of volume on the pressure of a given amount of a confined gas is true in general.

Decreasing the volume of a contained gas will increase its pressure, and increasing its volume will decrease its pressure. In fact, if the volume increases by a certain factor, the pressure decreases by the same factor, and vice versa. Volume-pressure data for an air sample at room temperature are graphed in . If a gas has an initial pressure of 24,650 Pa and an initial volume of 376 mL, what is the final volume if the pressure of the gas is changed to 775 torr?

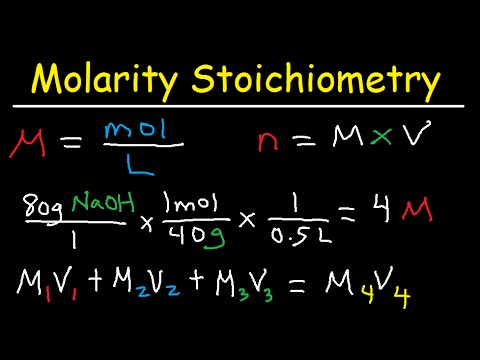
Volume is the level at which something is heard or the amount of space a solid, liquid or gas occupies. With a container, its volume would be its capacity, or how much it can hold. Volume is often expressed in cubic units determined by the International System of Units. The volume units must be the same for both volumes in this equation. In general, M1 usually refers to as the initial molarity of the solution.